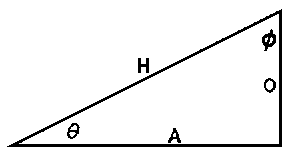
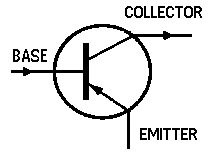
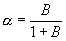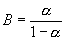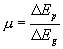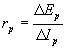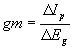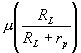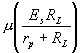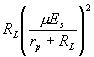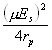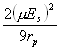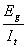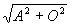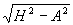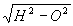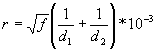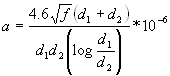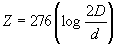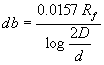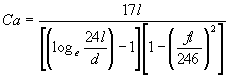Using Transistors Only
| In comparing the PNP and NPN circuits | this interchange in the transistor, circuits | |||||
| shown here, note that the current flow in the components of one is completely re- versed in the other. With the vacuum tube, this complete interchange of current and voltage polarities does not exist. Because of |
which have no parallel in vacuum-tube circuitry can be produced. Nevertheless, the circuits of transistorized equipment are still auite similar in many respects to those of equipment employing vacuum tubes. |
|||||
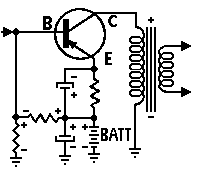
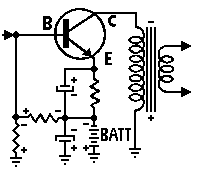
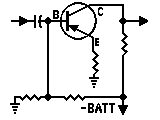
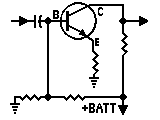
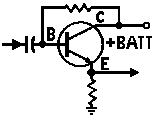
| Using PNP Transistors | ||
 |
 |
|
| With Positive Battery Terminal Grounded |
With Negative Battery Terminal Grounded |
|
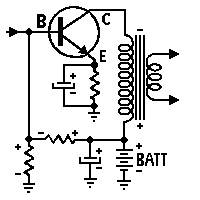
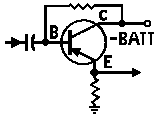
| Using NPN Transistors | ||
 |
 |
|
| With Positive Battery Terminal Grounded |
With Negative Battery Terminal Grounded |
|

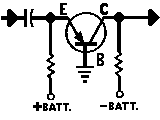
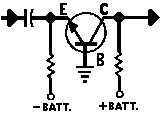

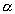
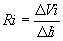
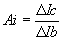 (with Vc constant)
(with Vc constant)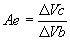 (with Ic constant)
(with Ic constant)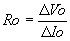
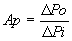
 (with Vc constant)
(with Vc constant)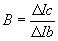 (with Vc constant)
(with Vc constant)